New model of the profit split in the pool
All players receive same percentage of expected value
The example
| CFC | HyperEV, $ | Profit, $ | EV diff |
|---|---|---|---|
| 80% | 1000 | 800 | -20% |
| -1000 | -1200 | -20% | |
| 110% | 1000 | 1100 | 10% |
| -1000 | -900 | 10% |
Pool profit calculator
| Player | |
|---|---|
| Room | |
| Stake | |
| Tournament | |
| ChipEV | |
| Net Won | |
| Pool | |
|---|---|
| Profit | $ |
| EV | $ |
| BI | $ |
| Scam | $ |
| Added cash | $ |
Advantages
Split profit even if EV is negative
No matter if your EV is negative or positive,
you split profit by CFC in any cases
Fast compensation of downswing
The player who finished with negative EV
and has downswing receive compensation immediately
Fixed EV-freerolling
Loosing EV in upswing to earn rakeback become unprofitable.Players have responsible attitude to the game.
Less exploitable
In models with deferred split until EV become positive the players who have information
about deferred profit receive an advantage. They can leave the pool and shift compensation
of downswings to the permanent and new participants. This does not make sense in our model.
Calculations
Profit is calculated by the following formulas:
Calculate CFC
| EV | Net Won | |
| Players with EV>0 | ||
| Players with EV<0 |
FAQ
The more CFC is, the more is compensation. But if downswing is a small percentage of EV and CFC is low, the compensation to the pool is possible.
For example, EV is -100$, loss -120$:
| CFC | Payment |
|---|---|
| 100% | 20$ |
| 90% | 10$ |
| 70% | -10$ |
Loss would be compensated by HS. The amount would become player’s personal make-up.
For example. EV is -100$, loss -80$:
| CFC | Payment | Mke-up |
|---|---|---|
| 130% | 10$ | 70$ |
| 100% | -20$ | 100$ |
| 80% | -40$ | 120$ |
The players with negative EV and upswing will pay to the pool the difference until their minus by EV, considering CFC.
For example, if EV is -100$, Net Won 50$, with CFC 80% the player pay to the pool 170$: 150$ upswing and 20$ more.
EV− и P− — Negative EV and profit of players with negative EV after split.
N− и N+ – Downswings of players with negative and positive EV. We set the points on $ axis P+, P−, EV+ and EV−
As the pool has downswing, the point P+ is to the left of EV+, and P− – is to the left of EV−:
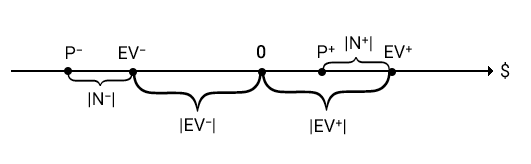
All players receive same percentage of expected value:
Let’s take the values of downswings and open the signs of the modul:
Profit of all players with negative and positive EV is total winnings of the pool (Net won):
Let’s express P− and P+ hrough NetWon and put into formula (1):
Multiply both sides of equation by (EV−*EV+):
Similarly
We’ve found total profit of players with negative and positive EV. Profit of a single player will be:
Let’s put the values of P+ and P− from (2). We get:
Relation
is called CFC of the pool
Without restriction of generality we prove that profit P+ of the players with positive EV are equal to their EV+, if the quantity of tournaments n→ ∞.
If,
So




